剥離を伴った任意翼型まわりの流れの解析的計算法
この記事の目的は、”とにかく解析的に”剥離を伴う翼の圧力分布を求めたい、ということです。
剥離を伴う流れを比較的解析的に計算する方法としては、境界要素法、自由流線理論、離散渦法、などが挙げられますが、結局いずれにせよ計算段階でパネル分割することになることがほとんどです。(最後まで解析的な導出法は見たことがない)
試行錯誤を繰り返すうちに、解析的に解くための条件は、(1)ポテンシャル流れであること、(2)単独翼であること、(3)境界条件が明確であること、と分かってきました。
そこで、本方法では剥離領域の排除効果を吹き出し流れによって近似することで導出します。(”wake source method”)*1
・さっそく導出してみる
最終的に翼面上の局所流速を
とすることで、流れの項を翼型の項から分離して導出する
複素速度ポテンシャルを周りで(
で発散しないように)展開すると
半径方向速度は
ここで、単位円上()の垂直速度分布に境界条件として吹き出し分布
を与えフーリエ級数展開することで係数比較をする
,
,
複素速度ポテンシャルに係数を戻して
速度場を実部(接線速度分布)と虚部(垂直速度分布)に分ける
ここではフーリエ級数の余弦と正弦を入れ替えた関数となっているが、これは”共役フーリエ級数”であり今井功先生の「等角写像とその応用」によれば次のように変換できる
よって、求めるべき局所流速分布は次のようになる(意外とシンプルな式にまとまった)
は循環成分であり、クッタの条件により決まる
剥離点と再付着点
を持つ剥離泡を近似するには、流れ関数が一定の時(
)流線が一致するので
・計算例
以下のグラフは入力した吹き出し分布と出力した共役フーリエ級数の比較です。

剥離泡の流速への影響が剥離領域に限られていることがわかります。「short bubble は翼型全体にわたる potential flow としての圧力分布にはあまり影響を与えない」*2という経験則と良い一致を示しています。
また、 圧力分布は次のようになりました。
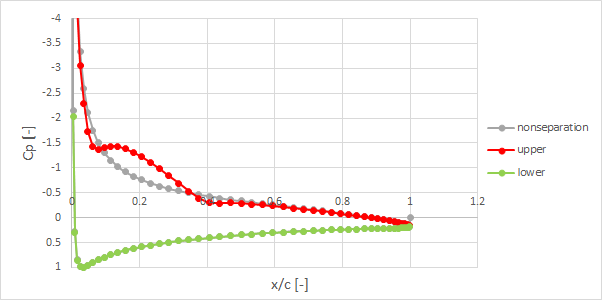
注意しなくてはいけないのが、いかにも剥離泡の特性である圧力一定領域と圧力回復を示しているように見えますが、あくまでも排除効果を利用した計算であり、この剥離領域においては特に意味のあるものではないです。
(吹き出し分布と逆流域の関係は考察中です)